Knot Physics
A geometric approach to unification
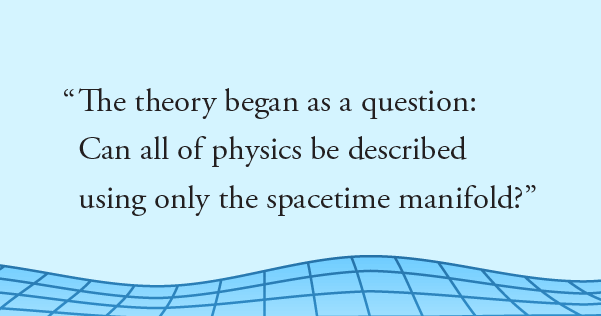
A geometric approach to unification
Theory Summary
An overview of the entire theory, from simple assumptions about the spacetime manifold through particles, quantum mechanics, and forces
Learn more
Theory Summary
An overview of the entire theory, from simple assumptions about the spacetime manifold through particles, quantum mechanics, and forces
Learn more
Papers
The papers cover theory fundamentals as well as a variety of topics, including entanglement and dark matter.
Read more
Latest Paper
Incorporating Gravity into the Path Integral of Quantum Mechanics Using the Thermodynamics of Spacetime
We use principles from the thermodynamics of spacetime to modify the path integral of quantum mechanics. Entropy of the vacuum is interpreted as microstates... Read more
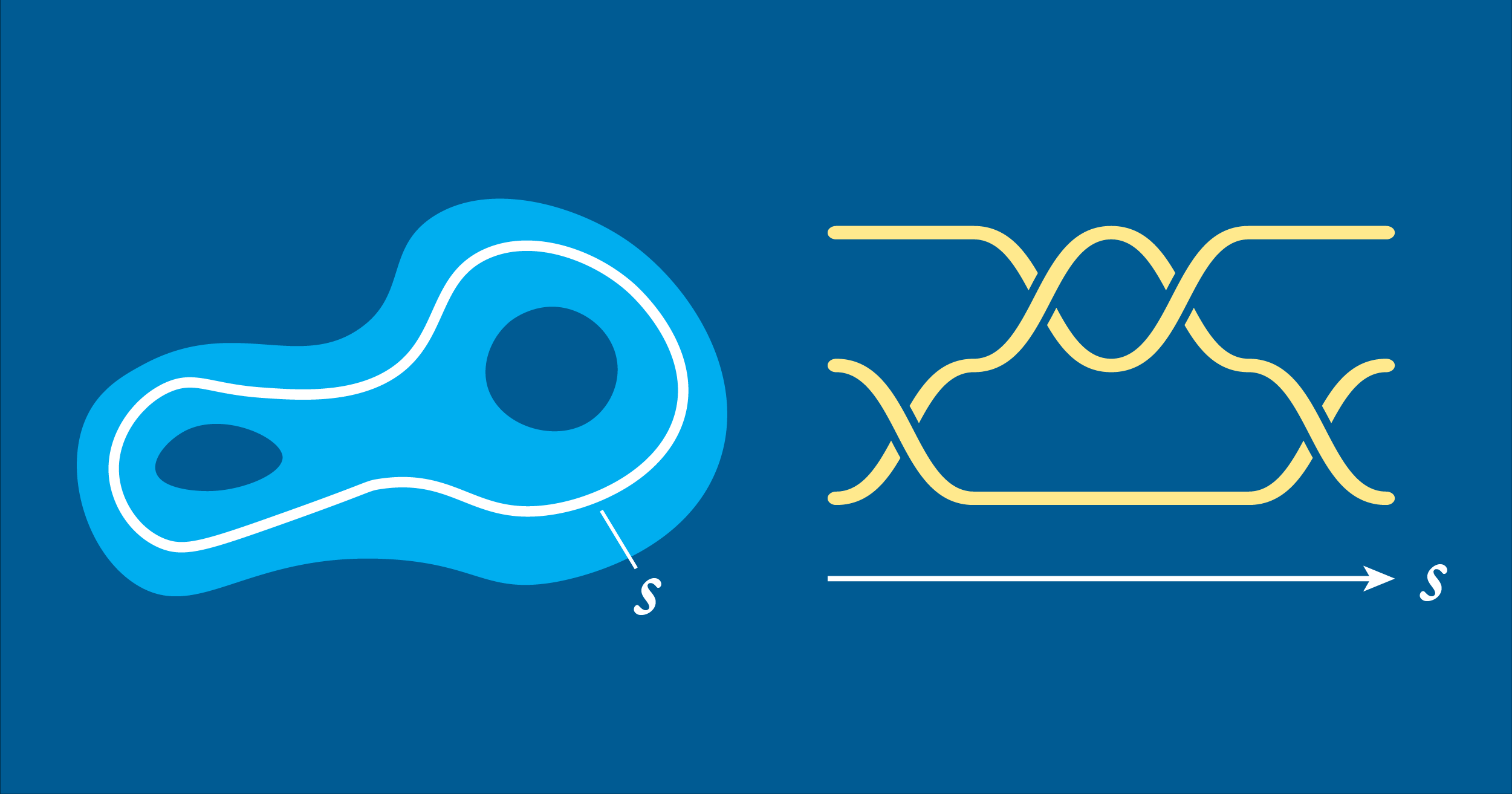
Topics in Knot Physics
More Resources
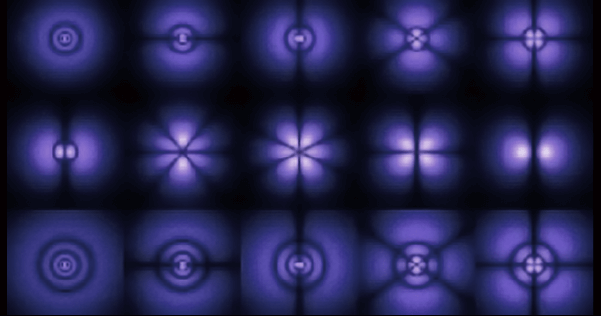
Quantum Mechanics
A 3-minute video on quantum mechanics, which results from a geometric model of spacetime
Quantum Mechanics
A 3-minute video on quantum mechanics, which results from a geometric model of spacetime